Spin and Valley Physics in Two Dimensional Systems Graphene and Superconducting Transition Metal Dichalcogenides

Doctoral Thesis Defense

evansosenko.com/deck-doctoral-thesis

August 2, 2016

Background

Graphene — By AlexanderAlUS (Own work) [CC BY-SA 3.0 or GFDL], via Wikimedia Commons

2D Materials promise novel application

Graphene discovered 2004
2D hexagonal carbon lattice, high electron mobility
Relativistic low-energy model: Dirac cones, linear dispersion
Strong, flexible & transparent
Monolayer TMDs: also hexagonal lattice
Gapped with strong spin-orbit coupling
Both excellent candidates for spintronic devices

Spintronics

Nobel Prize for GMR in 2007 (Albert Fert, Peter Grünberg)
A future with devices built on spin-current
Smaller and lower power
Graphene? Predicted excellent conductor for spin-current
TMDs? No spin degeneracy and spin couples to light polarization

Overview for Part I

Spin lifetime

Injection, transmission, detection of spin signals
Spin signals degrade via internal scattering
Spin lifetime measures how quickly this happens
Critical to find materials with long spin lifetimes

Motivation

Theoretical predictions longer than measured: $\text{ms}$ vs. $\text{ps}$
Finite contact resistance mismatch: a potential candidate
Unified analytic solution for fitting data in all limits

Outline

Model and solution
Hanle curve fitting
Regimes and results

E. Sosenko, H. Wei, and V. Aji, Phys. Rev. B 89, 245436 (2014).

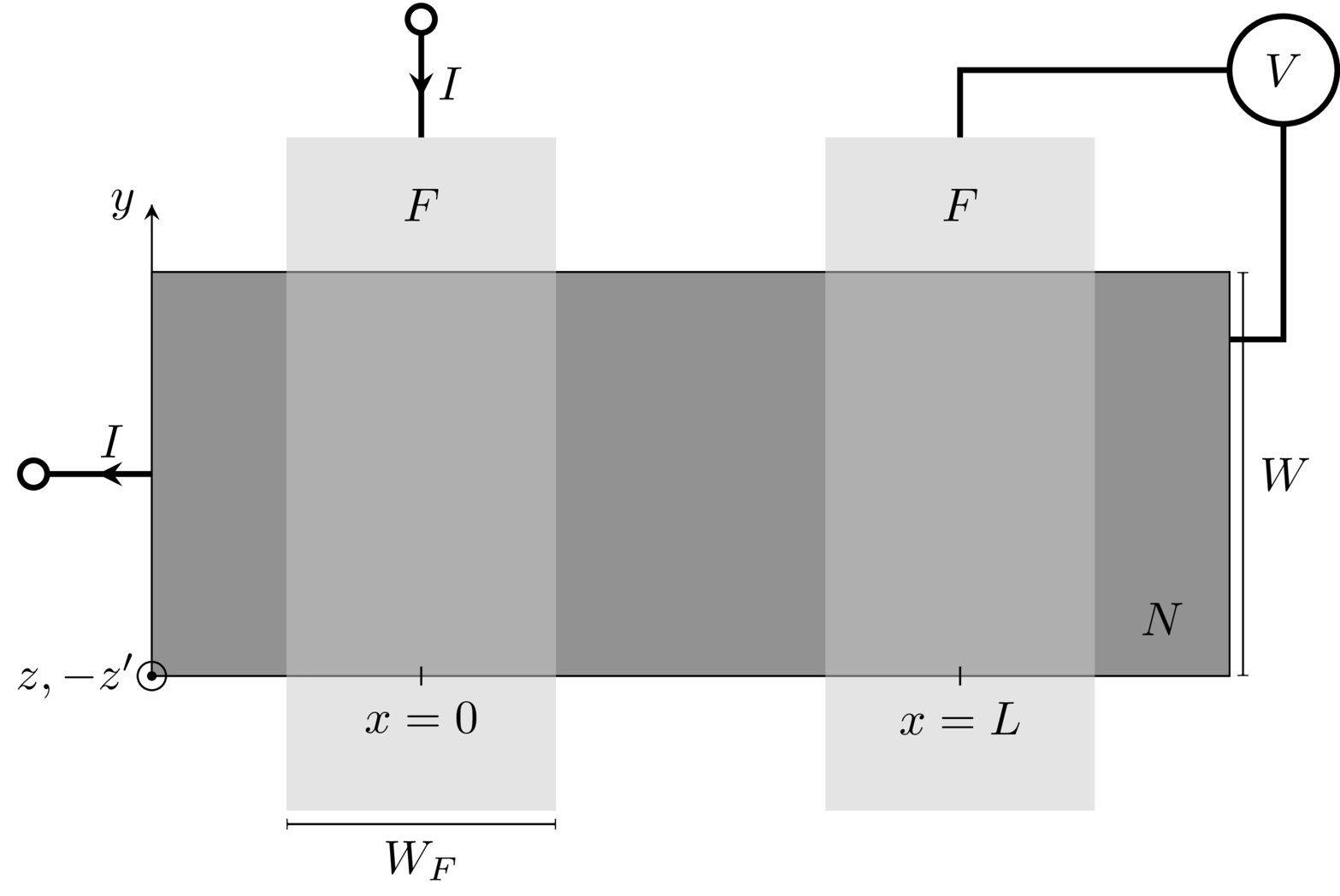
Device geometry

$L$ : contact spacing
$D$ : diffusion constant
$\tau$ : spin lifetime
$\lambda = \sqrt{D \tau}$
$\omega = g \mu_B B / \hbar$

$\mu_s = \frac{1}{2} \left( \mu_{\uparrow} - \mu_{\downarrow} \right)$
$J_{\uparrow \downarrow} = \sigma_{\uparrow \downarrow} \nabla \mu_{\uparrow \downarrow}$
$J_{\uparrow \downarrow}^C = \Sigma_{\uparrow \downarrow} \left( \mu^N_{\uparrow \downarrow} - \mu^F_{\uparrow \downarrow} \right)_c$
$J = J_{\uparrow} + J_{\downarrow}$
$J_s = J_{\uparrow} - J_{\downarrow}$

$D \nabla^2 \mu_s - \frac{\mu_s}{\tau} + \omega \times \mu_s = 0$

$V \propto \mu_s^N(x = L)$

$R_\text{NL} = V / I$

J. Fabian, A. Matos-Abiague, C. Ertler, P. Stano, and I. Žutić, Acta Physica Slovaca 57, 565 (2007). M. Johnson and R. H. Silsbee, Phys. Rev. B 37, 5312 (1988). S. Takahashi and S. Maekawa, Phys. Rev. B 67, 052409 (2003). M. Popinciuc, C. Józsa, P. J. Zomer, N. Tombros, A. Veligura, H. T. Jonkman, and B. J. van Wees, Phys. Rev. B 80, 214427 (2009).

Motivation for solution

Existing results

All existing analytic expressions ignore contact resistance
Assume infinite resistance for convenience
Integral form widely used but difficult to fit
Only a numeric treatment of finite contact resistance

$R_{\text{NL}} \propto \text{Re} {\frac{e^{- \left( L / \lambda \right) \sqrt{1 + i \omega \tau}}} {2 \sqrt{1 + i \omega \tau}}}$

$R_{\text{NL}} \propto \int_0^{\infty} \frac{1}{\sqrt{4 \pi D t}} \exp{\left[ - \frac{L^2}{4 D t} \right]} e^{-t / \tau} \cos{\omega t} \: dt$

Our approach

Finite contact resistance
Exact analytic expression
Matches previous approaches in appropriate limits

Computation

Drift diffusion equation in contacts and semiconductor
Continuity of current and spin current at boundary
Conservation of current and spin
Solution gives nonlocal resistance

Non-local resistance

$\Delta R_{\text{NL}} = 2 P^2 R_N \left\lvert f \right\rvert$

$r = \frac{R_F + R_C}{R_{\text{SQ}}} W$
$R_{\text{SQ}} = W / \sigma^N$
$R_N = \frac{\lambda}{W L} \frac{1}{\sigma^N}$

$f = \text{Re} \left\{ \left( 2 \left[ \sqrt{1 + i \omega \tau} + (\lambda / r) \right] e^{\left( L / \lambda \right) \sqrt{1 + i \omega \tau}} + (\lambda / r)^2 \frac{ \sinh{\left[ \left( L / \lambda \right) \sqrt{1 + i \omega \tau} \right]}} {\sqrt{1 + i \omega \tau}} \right)^{-1} \right\}$

Only scales that appear in $f$

$L / \lambda$
$\lambda / r$
$\omega \tau$

E. Sosenko, H. Wei, and V. Aji, Phys. Rev. B 89, 245436 (2014).

Fits

Tunneling contacts

Fit to parallel field data from Fig. 4a of W. Han, et al.

$L = 2.1 \: \mu \text{m}$
$P = 0.19 \:$
$R_\text{C} = 2.03 \times 10^7 \: \text{k} \Omega$
$\tau = 514.3 \: \text{ps}$
$D = 0.02 \: \text{m}^2 \text{s}^{-1}$

Tunneling contacts

Fit to parallel field data from Fig. 4b of W. Han, et al.

$L = 5.5 \: \mu \text{m}$
$P = 0.1 \:$
$R_\text{C} = 6.70 \times 10^6 \: \text{k} \Omega$
$\tau = 451.84 \: \text{ps}$
$D = 0.01 \: \text{m}^2 \text{s}^{-1}$

W. Han, K. Pi, K. M. McCreary, Y. Li, J. J. I. Wong, A. G. Swartz, and R. K. Kawakami, Phys. Rev. Lett. 105, 167202 (2010).

Fits

Pinhole contacts

Fit to parallel field data from Fig. 4c of W. Han, et al.

$L = 3 \: \mu \text{m}$
$P = 0.23 \:$
$R_\text{C} = 2.31 \times 10^7 \: \text{k} \Omega$
$\tau = 132.28 \: \text{ps}$
$D = 0.02 \: \text{m}^2 \text{s}^{-1}$

Transparent contacts

Fit to parallel field data from Fig. 4d of W. Han, et al.

$L = 3 \: \mu \text{m}$
$P = 0.01 \:$
$R_\text{C} = 2.94 \: \text{k} \Omega$
$\tau = 130.36 \: \text{ps}$
$D = 0.04 \: \text{m}^2 \text{s}^{-1}$

W. Han, K. Pi, K. M. McCreary, Y. Li, J. J. I. Wong, A. G. Swartz, and R. K. Kawakami, Phys. Rev. Lett. 105, 167202 (2010).

Regimes

Zero field

$\Delta R_{\text{NL}} = \left( P_{\Sigma}^L \right)^2 R_N e^{- L / \lambda}$

Tunneling contacts

$f^{\infty} = \text{Re} {\frac{e^{- \left( L / \lambda \right) \sqrt{1 + i \omega \tau}}} {2 \sqrt{1 + i \omega \tau}}}$

Fits independent of lifetime

$\lambda / r \gg \sqrt{\omega \tau} \gg 1$

Zeros determined by $L \sqrt{\frac{D}{2 \omega}} + \frac{\pi}{4} = \frac{n \pi}{2}$

E. Sosenko, H. Wei, and V. Aji, Phys. Rev. B 89, 245436 (2014).

Fits: $\tau$ Independent Limit

Transparent contacts

$L = 3 \: \mu \text{m}$
$P = 0.02 \:$
$R_\text{C} = 0.27 \: \text{k} \Omega$
$\tau = 9.97 \times 10^9 \: \text{ps}$
$D = 0.02 \: \text{m}^2 \text{s}^{-1}$

Transparent contacts

$L = 3 \: \mu \text{m}$
$P = 0.02 \:$
$R_\text{C} = 0.28 \: \text{k} \Omega$
$\tau = 9.95 \times 10^{13} \: \text{ps}$
$D = 0.02 \: \text{m}^2 \text{s}^{-1}$

W. Han, K. Pi, K. M. McCreary, Y. Li, J. J. I. Wong, A. G. Swartz, and R. K. Kawakami, Phys. Rev. Lett. 105, 167202 (2010).

Software

Data Fitting with SciPy

Independent Python package for general curve fitting

github.com/razor-x/scipy-data_fitting

Fitalyzer

Browser based fitting visualizer

github.com/razor-x/fitalyzer

Transition metal dichalcogenides

TMD overview and tight-biding, low-energy, two-valley model
Optical valley selection rules and Berry curvature
Intrinsic and induced superconductivity
Intervalley and intravalley paring channels
Superconducting optoelectronics and Berry curvature

TMD monolayers

Direct band gap semiconductors: $\mathrm{MoS_2}$ , $\mathrm{WS_2}$ , $\mathrm{MoSe_2}$ , $\mathrm{WSe_2}$
Break inversion symmetry ⇒ gapped out spectrum
Two inequivalent valleys ⇒ new degree of freedom
Strong spin-orbit coupling ⇒ large valance band spin-splitting
Opposite valley Berry curvature
Optical valley probe and valley Hall effect
Two state tight binding model: $d_{z^2}$ , and $d_{xy} \pm i d_{x^2 - y^2}$

$H_{\tau}^0 \left( \mathbf{k} \right) = a t \left(\tau k_x \sigma_x + k_y \sigma_y \right) \otimes I_2 + \frac{\Delta}{2} \sigma_z \otimes I_2 - \lambda \tau \left(\sigma_z - 1 \right) \otimes S_z$

D. Xiao, G.-B. Liu, W. Feng, X. Xu, and W. Yao, Phys. Rev. Lett. 108, 196802 (2012).

Energy bands

$\Delta$ —band splitting
$\lambda$ —spin splitting
$\tau$ —valley index
$s$ —spin index

$a t = 3.15$ Å eV
$\Delta = 1.66$ eV
$2 \lambda = 0.15$ eV
$\mu = -0.83$ eV

E_{\tau \sigma}^n \left( \mathbf{k} \right) = \frac{1}{2} \left( \lambda \tau \sigma + n \sqrt{(2 a t)^2 {\left\lvert \mathbf{k} \right\rvert}^2 + {\left( \Delta - \lambda \tau \sigma \right)}^2} \right)

Optical excitations

H^A = \sum_{\mathbf{k}, \tau, s, n, n'} \frac{e A_0}{m_0} \mathbf{\epsilon} \cdot \mathbf{P}_{\tau s}^{n n'} \left( \mathbf{k} \right) {c_{\tau s}^n}^{\dagger} \left( \mathbf{k} \right) c_{\tau s}^{n'} \left( \mathbf{k} \right)

Optoelectronic coupling

Right circularly polarized light couples to right valley $P_+$
Left circularly polarized light couples to left valley $P_-$

Valley Hall effect

Semiclassical equations

$\dot{\mathbf{r}} = \nabla_{\mathbf{k}} E \left( \mathbf{k} \right) - \dot{\mathbf{k}} \times \mathbf{\Omega} \left( \mathbf{k} \right)$

$\dot{\mathbf{k}} = -e \mathbf{E} - e \dot{\mathbf{r}} \times \mathbf{B}$

Anomalous velocity

$\mathbf{v} = e \mathbf{\Omega} \left( \mathbf{k} \right) \times \mathbf{E}$

Berry curvature

$\mathbf{\Omega}_{\tau \sigma}^n \left( \mathbf{k} \right) = \nabla_{\mathbf{k}} \times \left\langle u_{\tau \sigma}^n \left( \mathbf{k} \right) \right\rvert i \nabla_{\mathbf{k}} \left\lvert u_{\tau \sigma}^n \left( \mathbf{k} \right) \right\rangle$

Broken inversion symmetry

$\mathbf{\Omega}_{\tau, \sigma}^n \left( \mathbf{k} \right) = - \mathbf{\Omega}_{-\tau, \sigma}^n \left( \mathbf{k} \right) \neq 0$

Superconducting sources

Substrate induced

$V = - \sum_{\mathbf{k} \nu \tau} B_{\nu} {d^{\nu}_{\tau}}^{\dagger} \left( \mathbf{k} \right) d^{\nu}_{-\tau} \left( \mathbf{k} \right)$

Intrinsic (density-density interaction)

$V = \frac{1}{2} \sum_{\mathbf{R} \mathbf{R'}} v_{\mathbf{R} \mathbf{R'}} :n_{\mathbf{R}} n_{\mathbf{R'}}:$

Assume even pairing interaction

BCS Hamiltonian

$H - \mu N = \sum_{\mathbf{k} \sigma} \xi_{\mathbf{k}} c_{\mathbf{k} \sigma}^{\dagger} c_{\mathbf{k} \sigma} - \sum_{\mathbf{k}} \left( \bar{\Delta}_{\mathbf{k}} c_{-\mathbf{k} \downarrow} c_{\mathbf{k} \uparrow} + \Delta_{\mathbf{k}} c_{\mathbf{k} \uparrow}^{\dagger} c_{-\mathbf{k} \downarrow}^{\dagger} \right) = \sum_{\mathbf{k} \sigma} \lambda_{\mathbf{k}} \gamma_{\mathbf{k} \sigma}^{\dagger} \gamma_{\mathbf{k} \sigma}$

Superconducting channels

Induced

$\Delta_{\mathbf{k}} = \frac{1}{2} \left( B_+ + B_- \right) - \frac{1}{2} \left( B_+ - B_- \right) \cos{\theta_{\mathbf{k}}}$

Intrinsic

$\Delta_{\mathbf{k}} = \chi_0 \cdot f_{\mathbf{k}}$

Gap equation

$\chi_0 = \frac{v_0}{2} \sum_{\mathbf{k}} \frac{f_{\mathbf{k}} \cdot \chi_0}{\lambda_{\mathbf{k}}} \bar{f}_{\mathbf{k}}$

Project into upper-valance bands ( $\tau = s$ )
Mean field theory BCS-type solutions: gap function $\Delta_{\mathbf{k}}$
Different $f_{\mathbf{k}}$ per channel
$m = 0$ intervalley channel always dominates
Both classes give constant gap function for dominant paring

Optical coupling

$\mathbf{A} / A_0 = \mathbf{\epsilon} e^{i \omega t} + \mathbf{\epsilon}^* e^{-i \omega t}$ , $\mathbf{k} \rightarrow \mathbf{k} + e \mathbf{A}$
Harmonic perturbation ⇒ correlated phase's optical transition rates
Valley selection remains coupled to circularly polarized light

H^A = \sum_{\mathbf{k}, \tau, s, n, n'} \frac{e A_0}{m_0} \mathbf{\epsilon} \cdot \mathbf{P}_{\tau s}^{n n'} \left( \mathbf{k} \right) {c_{\tau s}^n}^{\dagger} \left( \mathbf{k} \right) c_{\tau s}^{n'} \left( \mathbf{k} \right)

Berry curvature

BCS ground state has zero net curvature
Optical pair-breaking ⇒ selectively excite carriers in single valley
Berry curvature retains relative sign relations

Conclusion

Spin lifetime measurements

Analytical solution modeling a nonlocal spin value which includes the ferromagnet contacts
Developed software to refit data with new result
Analyzed various regimes to understand the limits of the model

Superconducting states in TMDs

Characterized superconducting phases in regime where with locked spin and valley indexes
The correlated state inherits the valley contrasting phenomena
Pair-breaking produces quasiparticles that have the same Berry curvature (same anomalous velocity)

Background

2D Materials promise novel application

Spintronics

Overview for Part I

Spin lifetime

Motivation

Outline

Device geometry

Motivation for solution

Existing results

Our approach

Computation

Non-local resistance

Fits

Tunneling contacts

Tunneling contacts

Fits

Pinhole contacts

Transparent contacts

Regimes

Zero field

Tunneling contacts

Fits independent of lifetime

Fits: τ \tau τ Independent Limit

Transparent contacts

Transparent contacts

Software

Data Fitting with SciPy

Fitalyzer

Transition metal dichalcogenides

TMD monolayers

Energy bands

Optical excitations

Optoelectronic coupling

Valley Hall effect

Semiclassical equations

Anomalous velocity

Berry curvature

Broken inversion symmetry

Superconducting sources

Substrate induced

Intrinsic (density-density interaction)

BCS Hamiltonian

Superconducting channels

Induced

Intrinsic

Gap equation

Optical coupling

Berry curvature

Conclusion

Spin lifetime measurements

Superconducting states in TMDs

Fits: $\tau$ Independent Limit